End Behavior Of Rational Functions

Rational functions are mathematical entities that arise in various scientific and engineering applications, offering a means to describe and analyze relationships between quantities. This article aims to delve into the intricacies of rational functions, specifically focusing on their end behavior, which provides valuable insights into their behavior at extreme values of the independent variable.
Understanding Rational Functions
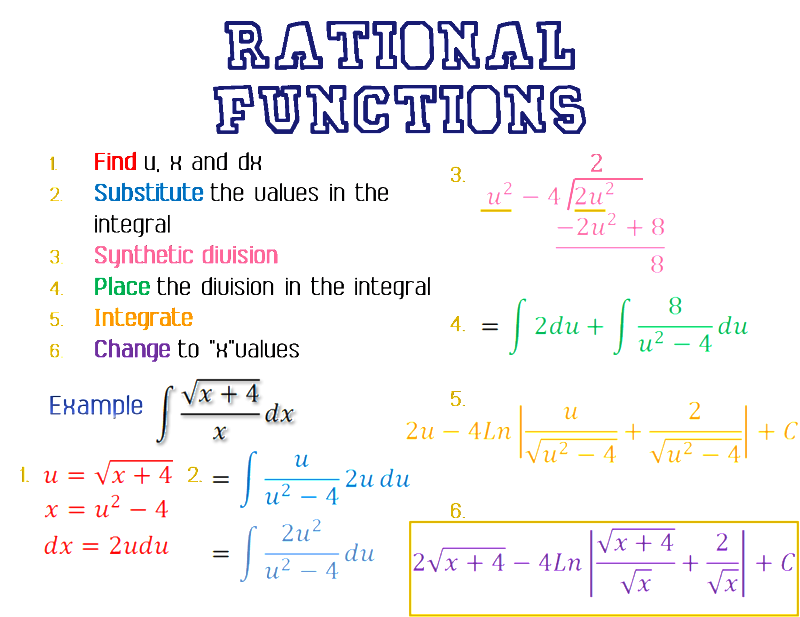
A rational function is a mathematical function defined as the ratio of two polynomial functions. In mathematical notation, a rational function f(x) can be expressed as:
f(x) = P(x) / Q(x)
where P(x) and Q(x) are polynomials, and Q(x) ≠ 0 to ensure the function is defined for all values of x except those that make the denominator zero.
Key Components of Rational Functions
Rational functions have two key components that influence their behavior and characteristics:
- Numerator: The polynomial P(x) in the numerator determines the function's general shape and behavior near its zeros.
- Denominator: The polynomial Q(x) in the denominator plays a crucial role in defining the function's end behavior and the existence of asymptotes.
End Behavior of Rational Functions

The end behavior of a rational function refers to how the function behaves as the independent variable x approaches positive or negative infinity. This behavior is influenced by the degrees and leading coefficients of the polynomials in the numerator and denominator.
Degree and Leading Coefficient of the Numerator
The degree of the numerator polynomial P(x) determines the overall growth rate of the function as x approaches infinity. If the degree of P(x) is greater than the degree of Q(x), the rational function will have a vertical asymptote at infinity. Conversely, if the degree of P(x) is less than the degree of Q(x), the rational function will have a horizontal asymptote at infinity.
The leading coefficient of P(x) influences the direction of the end behavior. If the leading coefficient is positive, the function increases without bound as x approaches infinity. Conversely, if the leading coefficient is negative, the function decreases without bound in the same limit.
Degree and Leading Coefficient of the Denominator
The degree of the denominator polynomial Q(x) plays a significant role in determining the end behavior of rational functions. If the degree of Q(x) is greater than the degree of P(x), the rational function will have a horizontal asymptote at y = 0 as x approaches infinity.
However, if the degree of Q(x) is equal to the degree of P(x)Q(x) is larger in absolute value than the leading coefficient of P(x), the rational function will have a horizontal asymptote at y = 0. On the other hand, if the leading coefficient of P(x) is larger, the rational function will have a slant asymptote.
Analyzing End Behavior: Examples
Let’s consider some specific examples to illustrate the end behavior of rational functions.
Example 1: f(x) = (x^2 + 3x - 2) / (x - 1)
In this case, the degree of the numerator is 2, and the degree of the denominator is 1. Since the degree of the numerator is greater, we expect a vertical asymptote at infinity. The leading coefficient of the numerator is positive, indicating that the function increases without bound as x approaches infinity.
Additionally, the denominator has a root at x = 1, which is a vertical asymptote. Thus, as x approaches 1 from either side, the function approaches positive or negative infinity, depending on the direction.
Example 2: g(x) = (x^3 - 2x^2 + x) / (x^2 + 1)
Here, the degree of the numerator is 3, and the degree of the denominator is 2. Since the degree of the denominator is greater, we expect a horizontal asymptote at y = 0 as x approaches infinity. The leading coefficient of the denominator is positive, indicating that the function approaches 0 from above as x becomes large.
Moreover, the denominator has no real roots, so there are no vertical asymptotes. As a result, the function remains bounded as x approaches infinity.
Applications and Real-World Scenarios
The study of rational functions and their end behavior has practical applications in various fields. For instance, in physics, rational functions can describe the behavior of objects under certain conditions, such as the motion of a projectile. In economics, rational functions can model supply and demand curves, helping to understand market behavior.
Additionally, rational functions are used in engineering to model signal processing systems, electrical circuits, and control systems. Understanding their end behavior is crucial for designing efficient and stable systems.
| Rational Function | End Behavior |
|---|---|
| f(x) = (x^2 + 3x - 2) / (x - 1) | Vertical asymptote at infinity; increases without bound as x → ∞ |
| g(x) = (x^3 - 2x^2 + x) / (x^2 + 1) | Horizontal asymptote at y = 0; approaches 0 from above as x → ∞ |
| h(x) = (2x^4 - 5x^2 + 3) / (x^3 - 2x) | Slant asymptote; increases without bound as x → ∞ |

FAQs
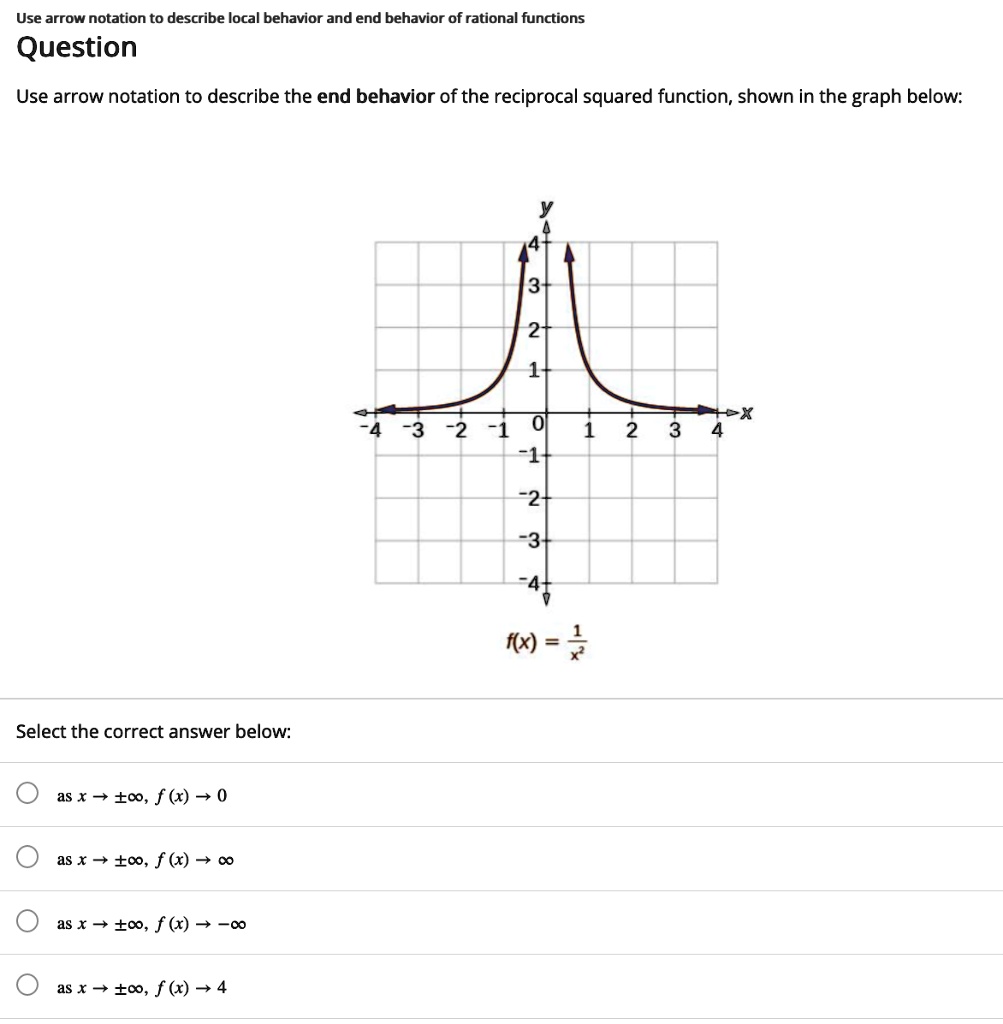
What are the key components that influence the end behavior of rational functions?
+
The end behavior of rational functions is primarily influenced by the degrees and leading coefficients of the polynomials in the numerator and denominator. The degree determines the overall growth rate, while the leading coefficient determines the direction of the end behavior.
How do rational functions behave at infinity?
+
The behavior of rational functions at infinity depends on the relationship between the degrees of the numerator and denominator polynomials. If the degree of the numerator is greater, there is a vertical asymptote at infinity. If the degree of the denominator is greater, there is a horizontal asymptote at y = 0. In the case of equal degrees, the leading coefficients determine the presence of a slant or horizontal asymptote.
Can rational functions have both vertical and horizontal asymptotes?
+
Yes, rational functions can have both vertical and horizontal asymptotes. Vertical asymptotes occur when the denominator becomes zero for specific values of x, while horizontal asymptotes are determined by the relationship between the degrees of the numerator and denominator polynomials.
What is the significance of understanding the end behavior of rational functions in real-world applications?
+
Understanding the end behavior of rational functions is crucial in real-world applications as it provides insights into the behavior of systems or phenomena at extreme values. This knowledge is essential for making predictions, designing efficient systems, and analyzing data in fields such as physics, economics, and engineering.


